Conjugate Heat Transfer Analysis on a graphics card.

Aim – Three dimensional CFD analysis of air cooling of a graphics card setup.
Introduction –
A Conjugate heat transfer (CHT) study has been performed for flow of air over a graphics card setup (consisting of a processor, fins and base plate) in Ansys Fluent.
The impact of inlet velocity on the average temperatures on the card has also been studied after performing a mesh refinement study.

Modelling and Solving approach –

Solid Model – A 3D model of basic graphics card setup was already provided. The card was enclosed in an enclosure to perform the study. The topology was shared using the Share feature of Spaceclaim to get conformal mesh between the solid and fluid regions. The geometry was then exported to Ansys Mesher. The section view of the geometry in Spaceclaim is shown below.

Meshing – Tetrahedral elements were used. Body sizing feature was used to mesh the graphics card parts separately.
Two different meshes were used for an inlet velocity of 1 m/s. The refined mesh was then used for inlet velocity of 2 and 3 m/s also.
The mesh details for both the cases are given in the below table.

The images of the mesh domain for case 1 and 2 are given below –

Case 1 – Baseline Mesh

Case 2 - Refined Mesh

Solver Settings – Ansys Fluent was used as the solver and steady state simulations have been performed using a pressure based solver.
Boundary conditions and materials – The boundary conditions can be understood from the below image. The front and back of the enclosure (not shown in the image) are also assigned symmetry type BC. Also, all the components of the graphics card have been assigned wall BC. Velocity inlet of 1, 2 and 3 m/s has been used for the study.

Three different solid materials have been used for the 3 components of the setup. Air was used as the fluid.
The solid materials and their properties have been defined in the below table. Only aluminium was available in the Fluent database. Rest were created manually.

Computational model – k-omega-SST model (Standard wall function) has been used since we are interested in the flow physics around the graphics card and this model is recommended for such problems. The energy equation has also been solved.
Since this problem involves multiple solid objects, special attention needs to given while setting up the problem. The fluid and solid cell zones must be properly assigned. Also, the processor has been considered as the volumetric energy source. Thus, source term needs to be defined in the cell zone conditions. A constant value of the source has been used. This value is obtained considering a TDP of 80 W. The heat generation rate q is given as

q=TDP/(Volume of processor) 

Also, fluent automatically splits the graphics card walls into wall/wall-shadow. It must be checked that proper materials have been assigned to these zones and also the walls are coupled.
Spatial Discretization Method – Coupled Pressure velocity coupling scheme was used to solve the Navier Stokes Equations. The default solution controls and methods have been used.

Results and Discussion –

All the parameters of interest (heat transfer coefficients, velocity, wall temperatures) are observed to be dependent on the flow velocities.
Average and peak temperatures decrease as the velocity is increased. This is because as the velocity is increased the heat transfer coefficient increases and thus, heat transfer due to convection also increases. This can be observed in both the heat transfer coefficient and wall temperature contours shown below.
Also, there is observed a separation zone beside the graphics card. This is because of the flow separation due to the vertical fins and base plate support lying normal to the flow direction. Also, the decrease in are of the flow above the graphics card results in increase of velocities in the domain.
The peak temperatures might not be realistic, however, the flow physics are definitely following the laws of nature indicating the correctness of the simulation study. High temperatures can be attributed to the material properties and high volumetric heat generation rate. To test this hypothesis, the volumetric heat generation rate is reduced by a factor of 10 and its effect is observed on the peak and average temperatures. It is concluded that the high volumetric heat generation rate is the main reason for such high temperatures and the solutions setup is correct.

The below graph shows the variation of the temperatures with increase in velocity for a constant heat generation rate. It can be clearly observed that the average and peak temperatures decrease with increase in velocity.

Convergence - The convergence criterion has been set 1e-03 for all the parameters except for energy for which the convergence criterion is 1e-06. Apart from the residuals, average temperature of the graphics card has also been observed to check for steady state.

The contours and other results for all the five cases given in the above table are shown below –

Case 1 –

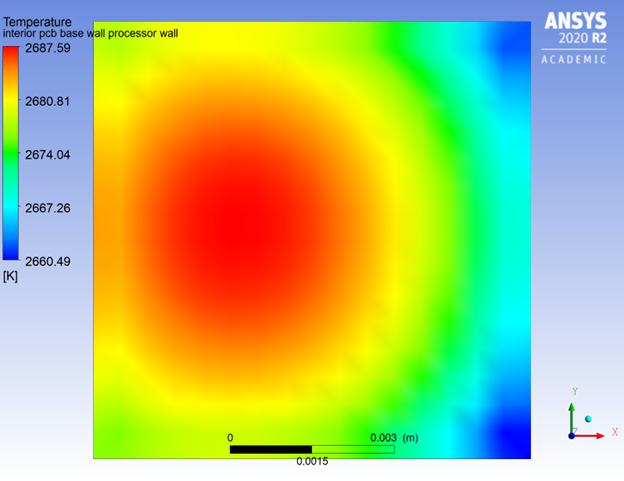
Case 2 - 

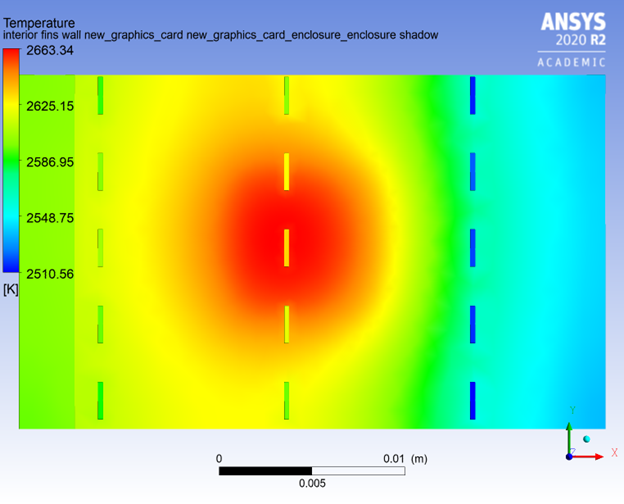
Case 3 -

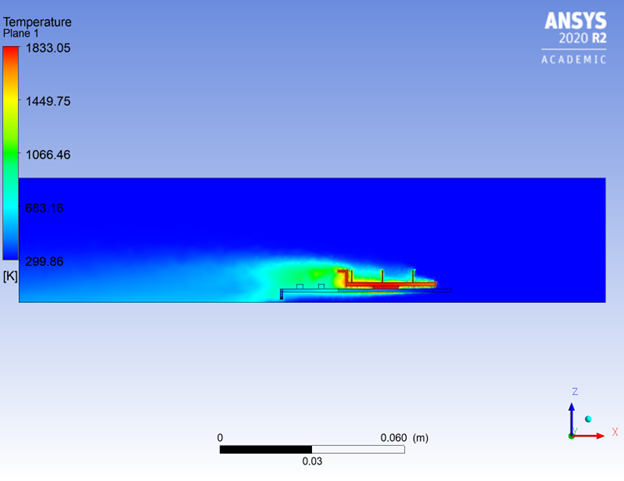
Case4 -

Case 5 -