Week 5 - Rayleigh Taylor Instability

Rayleigh Taylor Instability

Rayleigh Taylor Instability is an instability  of an interface between two fluids of different densities which occurs when the lighter fluid is pushing the heavier fluid.

As the RT instability develops, the initial perturbations progress from a linear growth phase into a non-linear growth phase, eventually developing plumes flowing upwards  and spikes falling downwards. In the linear phase, the fluid movement can be closely approximated by linear equations and the amplitude of perturbations is growing exponentially with time. In the non-linear phase, perturbation amplitude is too large for a linear approximation non-linear equations are required to describe fluid motions. In general, the density disparity between the fluids determines the structure of the subsequent non-linear RT instability flows.

Richtmyer–Meshkov instability 

The Richtmyer–Meshkov instability (RMI) occurs when two fluids of different density are impulsively accelerated. Normally this is by the passage of a shock wave. The development of the instability begins with small amplitude perturbations which initially grow linearly with time. This is followed by a nonlinear regime with bubbles appearing in the case of a light fluid penetrating a heavy fluid, and with spikes appearing in the case of a heavy fluid penetrating a light fluid. A chaotic regime eventually is reached and the two fluids mix. This instability can be considered the impulsive-acceleration limit of the Rayleigh Taylor Instability.

Kelvin–Helmholtz Instability

It occurs when there is velocity shear in a single continous fluids, or when there is a velocity difference across the interface between two fluids.The theory predicts the onset of instability and transition to turbulent flow in fluids of different densities moving at various speeds

Plateau–Rayleigh Instability

The Plateau–Rayleigh instability, often just called the Rayleigh instability, explains why and how a falling stream of fluid breaks up into smaller packets with the same volume but less surface area. It is related to the Rayleigh Taylor Instability and is part of a greater branch of fluid dynamics concerned with fluid thread breakup. 

The driving force of the Plateau–Rayleigh instability is that liquids, by virtue of their surface tension, tend to minimize their surface area. A considerable amount of work has been done recently on the final pinching profile by attacking it with self similar solutions

Objective 

To perform the Rayleigh Taylor instability simulation for 3 different mesh sizes.

1. Baeline Mesh
2. Refined Mesh
3. Finest Mesh

Geometry

Above is the geometry for the simulation with two phases named as air and water. 

1. Baseline Mesh 

Mesh

The following mesh contains an cell count of 3200 with an element size of 0.5 mm which is the baseline mesh.

Residuals

Above plot is the residuals for the current mesh which converged around 9500 iterations and from that time it started to converge for every 20 to 30 iterations.  

Contour of Volume Fraction

Initial

Above is the initial contour for the simulation where red is the water and blue one is the air.

Transitional

A

B

C

The contour which are shown up the transition period where the two phase begins to interfer with one another. The transition A is the startings of the interference creating a plumes region in the middle.The transition B shows an bubble region in the bottom phase of the simulation and at the top of the phase it begins to settle down.The transition C shows that the interference shifts to an almost finish phase.   

Final

From the above contour we can see that volume fractions are different when it is compared to initial stage meaning it has come to an halt with highest volume fraction at the bottom. 

Animation of the BaseLine Mesh

Case 1: Refined Mesh

Mesh

The Mesh has been refined with an element size of 0.3mm which give us a cell count of 8978.

 Residuals

The residuals was similiar to the baseline mesh but it converged around 10000 iterations and converged for every 20 iterations till the end of the timestep.

Contour of Volume Fraction

Initial

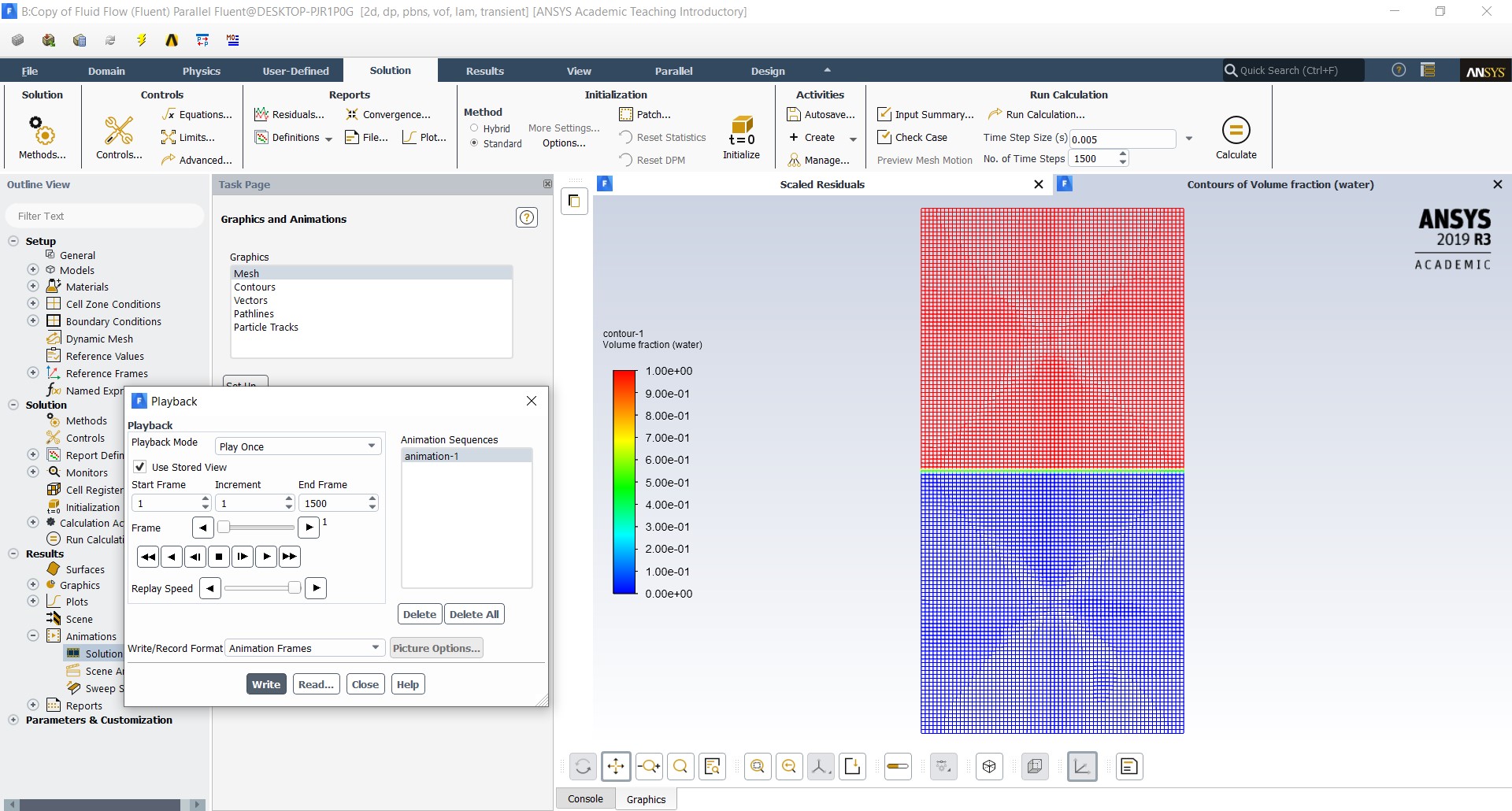
Above is the initial volume fraction contour with refined mesh with no difference in the phases.

Transitional

A

B

C

The contour which are shown up the transition period where the two phase begins to interfer with one another. The transition A is the startings of the interference creating a aggersive lumes region in the middle. The transition B shows an violent flame like region in the middle with some parts of bubble region in it. The transition C shows that the interference has come to an end.

Final

The final volume fraction contour shows a finished phase.

Animation of Refined Mesh

Case 2: Finest Mesh

Mesh

The Mesh has been refined with an element size of 0.15mm which gives a cell count of 35380.

Residuals 

The iterations has converged around 11000iterations

Contour of Volume Fraction

Initial 

Above is the initial volume fraction contour with refined mesh with no difference in the phases.

Transitional

A

B

C

This contours gives an clear transitions in the simlulations.

Final

The final volume fraction contour shows a finished phases.

Animation of Finest Mesh

Steady State

Steady state approach is not an viable option in this type of simulation beacuse this type of simulation is more time dependent but steady state appraoch is not. 

Atwood Number 

The Atwood number (A) is a dimensional number in fluid dynamics used in the study of hydrodynamic instabilities  in density stratified flows. It is a dimensionless density ratio.

where

 = density of heavier fluid

= density of lighter fluid.

For our case Atwood number is 0.997.

If Atwood number is equal to 0 RT instability flows take the form of symmetric “fingers” of fluid.

If Atwood number is equal to 1, the much lighter fluid “below” the heavier fluid takes the form of larger bubble-like plumes.